Shifting Patterns : July 2014

Thursday, 31 July 2014

the weaver bird

Weaver birds, also known as weaver finches, get their name because of their elaborately woven nests
(the most elaborate of any birds'), though some are notable for their selective parasitic nesting habits. The nests vary in size, shape, material used, and construction techniques from species to species.
(Source: Wikipedia)
Yay, for all the wonderful bird life in nature !

Monday, 21 July 2014

transport Egyptian fractions { keep on truckin' }

5/12 = 1/4 + 1/10 + 1/15 = 1/5 + 1/6 + 1/20

Lcm( 12, 4, 10, 15, 5, 6, 20 ) = 60

25/60 = 15/60 + 6/60 + 4/60 = 12/60 + 10/60 + 3/60

60 is the smallest number with 6 representations as a sum of 2 primes:

60 = 7+53 = 13+47 = 17+43 = 19+41 = 23+37 = 29+31

Thursday, 17 July 2014

gravity force ~ ^", '> ;-~

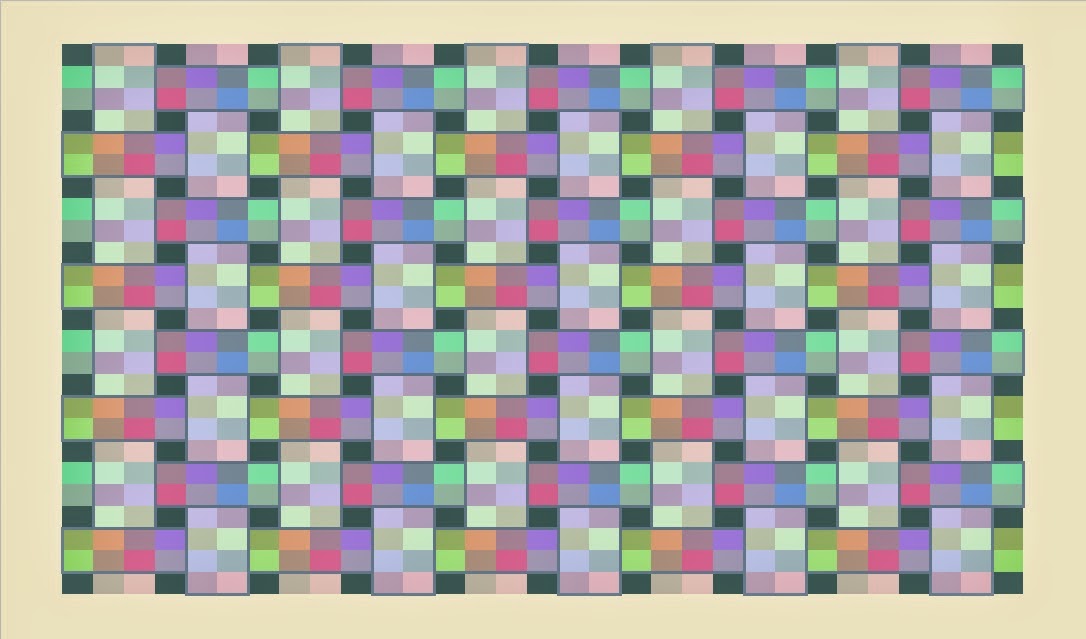
The dynamics of positive integers with regards to happy numbers

A happy number example
A number such as 334 is three hundred and thirty four with digits forming the multiset { 3, 3, 4 }
We then sum the squares of these digits: 3^2 + 3^2 + 4^2 = 9 + 9 + 16 = 34
Now we do the same for 34: 3^2 + 4^2 = 9 + 16 = 25
And iterating this process:
2^2 + 5^2 = 4 + 25 = 29
2^2 + 9^2 = 4 + 81 = 85
8^2 + 5^2 = 64 + 25 = 89
8^2 + 9^2 = 64 + 81 = 145
1^2 + 4^2 + 5^2 = 1 + 16 + 25 = 42
4^2 + 2^2 = 16 + 4 = 20
2^2 + 0^2 = 4 + 0 = 4
If we keep going there is a cycle:
4^2 = 16 ; 1^2 + 6^2 = 37 ; 3^2 + 7^2 = 58 ; 5^2 + 8^2 = 89 ; and then 145, 42, 20, 4, as before.
If the number enters into the cycle 4, 16, 37, 58, 89, 145, 42, 20, 4 the number is called “happy”.
The only other cycle is 1, 1, 1, 1, …
An interesting question is: how do happy numbers “enter into” the cycle ?
For example, with 334, the number enters into the cycle at 89.
This visualisation shows the dynamics of this process for numbers 1 up to 40 using distinctive colours for the digit values 0, 1, 2, …, 9 and box borders to show where the number enters into a cycle.