omega3

   three layers of information omega, bigomega, and the differences between these two values   

fractal sequence variations

The Jaded Dynamic Neutrosophic Stratosphere

  SOR~ ; \??/ ; ~10 >> BIG   

 

  SOR~ ; /?\ ; ~10   

turning the light on divisor chains

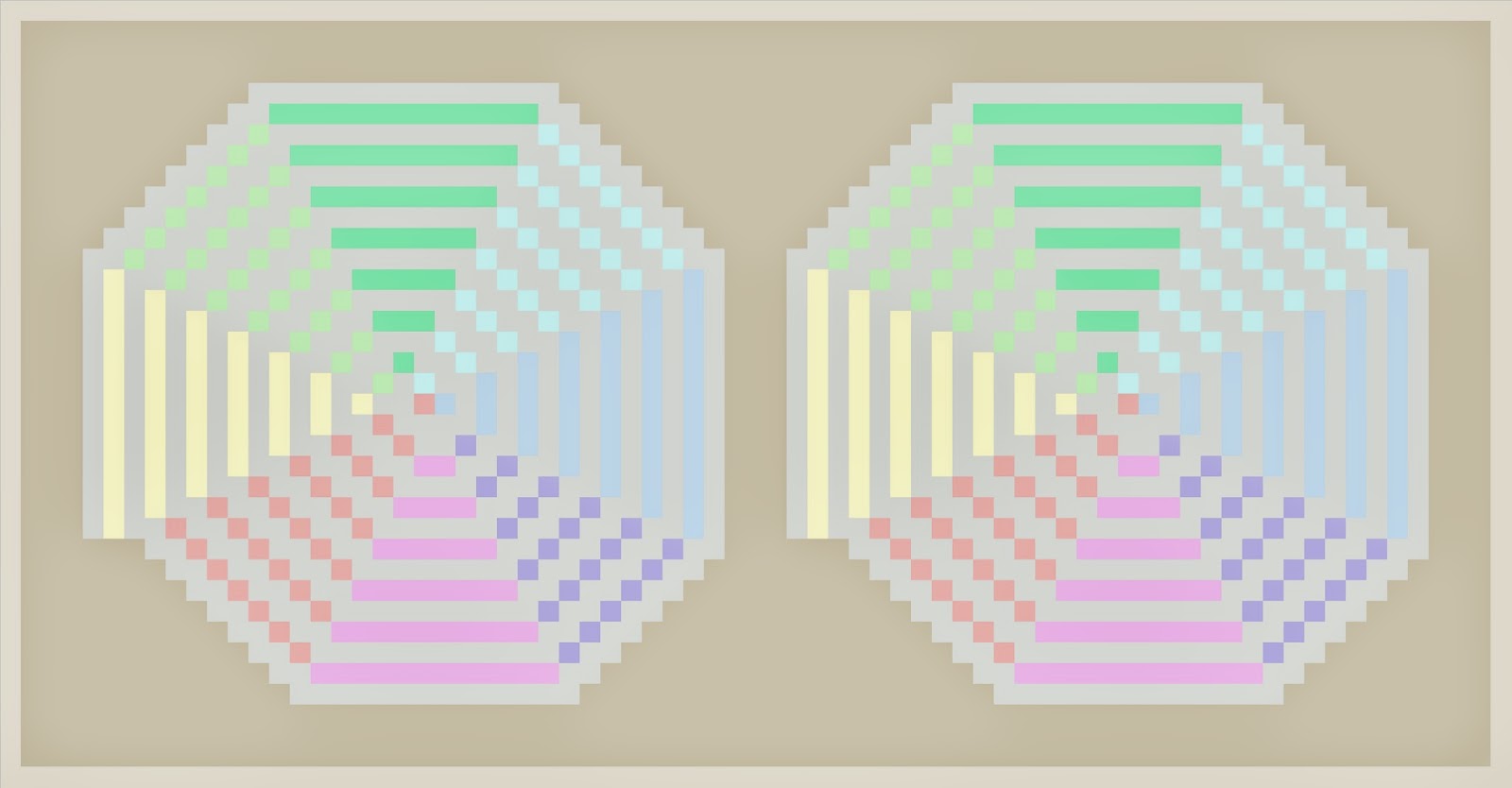
two similar art works based on the concept of divisor chains, a simple example to illustrate
start with a number, say 3.  clearly 3 | 3  ( 3 divides 3 )
3, 1  is OK as  1 | (3+1) ;
  3, 1, 2  is OK as  2 | (3+1+2)  ;
    3, 1, 2, 6  is OK as  6 | (3+1+2+6)
      3, 1, 2, 6, 4  is OK as  4 | (3+1+2+6+4)
        3, 1, 2, 6, 4, 8  is OK as  8 | (3+1+2+6+4+8)
the idea is to consider earliest ( smallest ) whole numbers not yet used
until we find a value is OK, satisfying the required property.
the sum is up to 24.  at this stage, adding 5 isn't going to help, neither is adding 7, nor 9,
nor 10, nor 11, what about 12 ?
12 does the job nicely as 24 + 12 = 36 and 12 | (3+1+2+6+4+8+12)
the puzzle is to discover from the grouping and ordering of the coloured rectangles
what are the divisor chains represented from permutations of { 1, ..., n }

Sieves

Even Sieve

Gradual Replacement Sieve

thinking with concepts

Lucas numbers and 33